- Head of Research Group: Prof. Mihály KOVÁCS
- Members of the Group: Mihály András VÁGHY
- Contact: kovacs.mihaly@itk.ppke.hu
- The mathematical model of many time- and space-dependent processfies is described by partial differential equations. If there is uncertainty in the equation, then the uncertainty can be modeled using stochastic partial differential equations. If a process taking place in a given location and/or at a given time instance is also affected by events further away in space and/or time, then the process can be described using non-local differential equations. The focus of the group's work is the mathematical theory and numerical analysis of the above equations. Recent research topics include the applications of stochastic partial differential equations to generate Gaussian random fields on metric graphs to provide input for various statistical models.
- The solution of these models require finite element solutions of elliptic problems on metric graphs which became large when the size of the network grows. To speed up the solution and lessen the memory requiremets one may emply domain decomposition methods which is also one of the current research focus of the group.
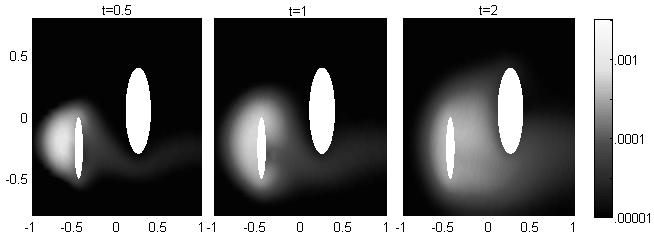
Fractional dispersion along flow lines around obstacles


